Two dates before the end of the 2018 World Cup Conmebol Qualifiers, I dared to predict, considering probability theory, the teams that would qualify. I obtained that Brazil, Uruguay, Argentina, Colombia and Peru were meant to do it, being the latter the one that would play the intercontinental playoffs. I invite you to read this analysis, where we grasp the concept of probability theory. A summary of this article with some extra info appears in the following video:

The Qualifiers are reaching the final stretch. Two dates are left and fans cannot stop seeing the standings over and over again. Each team has two matches left. There are 10 teams in total. How many results can arise? It seems that no so much, but maybe we are wrong. Each team can win, draw or lose. Three states! What's better, they come in pairs, if one team wins the other is bound to lose and if one draws the other is bound to draw. Sounds interesting: The state of one team automatically determines the state of the other. No! This is not quantum entanglement. Don't get confused. We are in the classical world, if you want to immerse yourself in the quantum world I invite you to read the article: "Quantum entanglement and the end of cybersecurity." Now we will just give a basic notion of probability theory.

If there are five games on one date and the results of each one can be 3 (win, lose or draw), how many combinations will there be? The answer is 3 x 3 x 3 x 3 x 3 = 243. That is, on the penultimate date there are 243 ways in which the standings can be. Wow…! That's not all, on the last date it will be the same. So how many combinations are there in total? The answer is 243 x 243 = 59,049. Yes, I know, the number is gigantic and we haven't considered the number of goals. Our goal is to reduce this number in order to simplify the analysis and thus easily determine the five teams that qualify (in this article, every time we talk about a qualified team, we take into account the team that qualifies to the intercontinental playoffs). Let's check the games:

Home		Away
Bolivia	vs	Brazil
Venezuela	vs	Uruguay
Chile	vs	Ecuador
Colombia	vs	Paraguay
Argentina	vs	Peru

Table I: Last two dates of the Qualifiers.

Ecuador	vs	Argentina
Uruguay	vs	Bolivia
Paraguay	vs	Venezuela
Brazil	vs	Chile
Peru	vs	Colombia

The match Bolivia vs Brazil, given in Table I, is of particular interest. Do you think the outcome of this match will alter the standings? Let's see:

Table II: Standings before the last two dates.

Position	Country	Points	GF	GA	GD
1		37	38	11	27
2		27	28	18	10
3		26	19	16	3
4		24	26	25	1
5		24	16	15	1
6		23	24	23	1
7		21	17	23	-6
8		20	24	24	0
9		13	14	34	-20
10		8	18	35	-17

Of course not! As you can see in Table II, Brazil is first and Bolivia almost last. So we can say that even if Bolivia wins 5-0, the standings won't change. Therefore, the penultimate date only has 4 important games. Following the calculations made initially we proceed:

Wow…! There are no longer 59,049 cases but 19,683. We are on the right track. What else can we do? Let's check the matches of Uruguay. It plays against Venezuela in Caracas and against Bolivia in Montevideo. Also, its goal difference (GD) is the highest (excluding Brazil) and it just needs to win one game to be in the World Cup... actually, it only needs one draw to qualify. It is feasible, in fact very easy to achieve. Therefore, we are going to assume without fear of being wrong that Uruguay is already qualified and its opponents, Bolivia and Venezuela, even if they win, won't change the standings. Therefore, on the penultimate date there are only 3 important matches and on the last one, only 4. We have:

Wow…! There are no longer 19,683 cases but 2,187. Among the remaining matches there is one that sets a before and after in the outcome of the Qualifiers. It's the match between Colombia and Paraguay. If we consider that anything can happen in that game, we would have to study 2,187 cases because Colombia, going third, could be left out of the World Cup. To simplify the analysis and thus ease the probability theory discussion, let's consider that Colombia wins in Barranquilla, then eliminates Paraguay and qualifies. If this is the case, then how many games are left to study? If Colombia wins, Paraguay's match against Venezuela on the last date is irrelevant, therefore, we only have two important matches on the penultimate date and three on the last one. This gives us:

That's right! If Colombia wins, we have 243 combinations left. Out of them, only one represents the end of the Qualifiers. In this analysis we already have three qualified teams: Brazil, Uruguay and Colombia. The candidates for the two remaining slots are Ecuador, Chile, Argentina and Peru. You must keep in mind that this is not a complete analysis because we are ignoring the possible victory of Paraguay or a possible draw against Colombia. We will discuss these cases later, for now let's focus on understanding the concept of probability theory... Having Ecuador, Chile, Argentina and Peru as the only candidates, then we must focus on their games, which are:

Home		Away
Chile	vs	Ecuador
Argentina	vs	Peru

Table III: The most important matches

Ecuador	vs	Argentina
Brazil	vs	Chile
Peru	vs	Colombia

As you can see in Table III, only 5 matches are important, that is, 3 x 3 x 3 x 3 x 3 = 243 possibilities. We are going to use probability theory to know, from a mathematical point of view, which teams are the favorite ones to qualify. Here we are not talking about favorites because in the last Qualifiers they qualified, or because they are now in a good position in the FIFA ranking, or because they play against weak teams in the last dates. No! Here we will formulate a simple analysis based on mathematics, therefore, the conclusions go beyond what intuition or belief can dictate. This is precisely how probability theory was born. Initially, it was believed that chance was set by a superior being, that if one rolled the dice and a six came out, it was because that number was meant to be. Over the years, mathematics overcame beliefs, men like Cardano, Galileo, Pascal and Fermat laid the first foundations of probability theory, which in a simple way says that the chances of an event to happen out of a total of N equally probable possible cases is equal to the ratio between the number of occurrences n of that event (favorable case) and the total number of possible cases, that is, n/N. Let's look at some simple examples considering the Qualifiers.

What is the probability that Argentina beats Peru and at the same time Chile beats Ecuador?

P() ∩ P() = 1/3 x 1/3 = 1/9 = 11.1%.

Since the only option a team has is to win, lose, or draw, then the probability of winning is 1 out of 3. And since the matches of Argentina and Chile are independent results, then the total probability is given by their multiplication, 1 out of 9. This number also tells us that there are nine ways in which Argentina vs. Peru and Chile vs. Ecuador can be grouped, which are:

Each sub-group (or set) is labeled with the letters A through I. Together they represent the possible outcomes of the penultimate date. WL indicates that the winner is the home team and the loser is the away team, DD indicates a draw, and LW indicates that the loser is the home team and the winner is the away team.

Two independent games always gives a probability of 1 out of 9. Now, what will happen on the last date? If we have 3 games, then we will have 3 x 3 x 3 = 27 possible consequences for each sub-group from A to I, giving a total of 27 x 9 = 243 combinations. All of them are shown in detail in the supplementary information.

What is the probability of getting A and then that Peru beats Colombia?

P(A) ∩ P() = 1/9 x 9/27 = 9/243 = 3.7%.

Since there are 9 sub-groups, then the probability of obtaining A is 1 out of 9. And since each sub-group has 27 possible consequences on the last date, then it is enough to count in how many of them Peru beats Colombia to get the second probability. It turns out to be 9, therefore, we have 9 out of 27, giving a total probability of 9 out of 243. In what follows, we show the most important conclusions that the probability theory yields:

To conclude with this study, we answer the following questions:

1. What is the minimum score needed to qualify

For this we will use the tree diagram. There are 9 sub-groups (A to I) and in each one we have the following probabilities (see Tables A.3, B.3, C.3, D.3, E.3, F.3, G.3, H.3 e I.3 in the supplementary information):

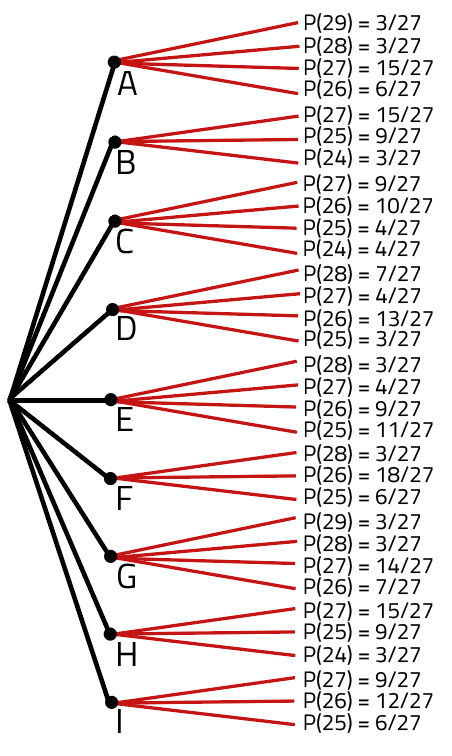
In Fig. 1., e.g., P(29) indicates the probability of obtaining 29 points in the last qualifying spot (fifth position in the standings).

This is known as a conditional probability because we want to find a value by conditioning it on one of the branches of the tree. Its result is given by the probability of getting A multiplied by the probability of getting 29 points in A. It is usually denoted P(A ∩ 29). Then, the probability of obtaining 29 points through all the branches would be the sum of them, i.e., P(29) = P(A ∩ 29) + P(B ∩ 29) + P(C ∩ 29) + P(D ∩ 29) + P(E ∩ 29) + P(F ∩ 29) + P(G ∩ 29) + P(H ∩ 29) + P(I ∩ 29). Doing this for different values ​​we get:

Therefore, the team in the last qualifying spot would likely have 26 or 27 points, i.e., P(26) = 75/243 = 30.9% and P(27) = 85/243 = 35%. This result is interesting because it allows us to define the minimum number of points that a team needs to qualify. For example, before playing the last two dates of the tournament, Uruguay already has 27 points with a goal difference of +10 and we said that it is enough for them to make one more point to qualify. If this happens, Uruguay would reach 28 points with the best goal difference. Therefore, we see that its probability of being eliminated is P(29) = 6/243 = 2.5%. The curious thing is that for this to happen, Chile must beat Brazil. Therefore, the fear of not getting one point in two games should not cause any concern to the Uruguayans.

Afternote: Once the contest finished, the fifth place had 26 points.

2. What happens if Paraguay draws with Colombia? And what happens if Paraguay wins?

As we have seen in the previous question, the minimum score in the last qualifying spot ranges between 24 and 29 points, which shows that Colombia, beating Paraguay, qualifies. In the event of a draw, Colombia would score 27 points (losing to Peru on the last date) and Paraguay would reach 25 (beating Venezuela on the last date). From all possible options given in the supplementary information, the best score possible for a non-qualified team (sixth position as much) is 28 points, but it only happens once out of 243 possibilities. It occurs when Argentina and Peru draw on the penultimate date and everyone, except Ecuador, wins on the last date, see Table D.1. In this case, Chile scores 29 points and Argentina and Peru 28 points, leaving Colombia out of the World Cup, but this event has a probability of 1/243 = 0.4%, that is, an event that is very difficult to happen. At this point in the analysis you could refute me by arguing that the 243 events that we are considering, only exist when Colombia beats Paraguay and it makes no sense to consider them for the case in which they draw. This is true; however, if we do the same analysis for the case of a draw, we will reach similar results to those given in the supplementary information, with the sole difference that now we will add Colombia and Paraguay in the standings; therefore, the 243 events described in this article give us a rough idea of ​​the qualifying probability of Colombia and Paraguay. To all this, if it is not fulfilled that Chile makes 29 points and Argentina and Peru 28 points, then would Colombia qualify with 27 points? Not necessarily, because there are 20 combinations out of the 243 possible ones in which the score of a non-qualified team is 27 points, that is, the same points as Colombia. Therefore, in this scenario, qualifying would depend strongly on the goal difference and luckily for the parceros they have one of the best goal differences in the standings. Consequently, Colombia's qualifying probability is very high even if it loses to Peru and draws with Paraguay.

On the other hand, Paraguay, drawing with Colombia and beating Venezuela, would reach a maximum of 25 points, with this score, it could fight for a slot in the World Cup. However, let us remember that Paraguay has a goal difference of -6, see Table II. To reduce this difference and have values ​​similar to the other teams, Paraguay is forced to thrash Venezuela 7-0. Something very difficult to imagine. For this reason, Paraguay, rather than fighting for the last qualifying spot, should target the fourth place, that is, see in how many cases the last qualifying spot is reached with 24 points in order to overcome it with its 25 points, i.e., Paraguay's chances are reduced to P(24) = 10/243 = 4%. A probability so low that it is better to accept the reality of saying goodbye to the World Cup. Due to the above discussion, a draw between Colombia and Paraguay does not greatly affect the results shown in this article.

And what happens if Colombia loses to Paraguay? In this case, before the last date, Paraguay accumulates 24 points and Colombia stays at 26. Similarly, since Paraguay has a goal difference of -6, they should focus on qualifying in the fourth place. If it stays at 24 points, it would be eliminated because there is no P(23). If it scores 25 points, that is, if it draws its last game against Venezuela, the conclusion would be identical to the previous case, i.e., its chances are 4%. Finally, by winning its last game, Paraguay would reach 27 points, which increases it probability to P(26)+P(25)+P(24) = 55%. Again, it should be noted that these results are approximations because we are studying 243 cases out of a total of 2,187. The funny thing is that we can infer that Paraguay, even if it wins its last two games, is at most close to 50% in its qualifying probability. On the contrary, Colombia, staying on 26 points, due to a very good goal difference, also obtains the same probability, i.e., P(26)+P(25)+P(24) = 55%. As you may notice, Colombia has better chances than Paraguay even when it loses its last two games. Therefore, it is feasible to think that the results presented here are sufficient to infer that Colombia has better chances of qualifying even when losing to Paraguay.

Afternote: Paraguay beat Colombia. This result did not affect the conclusions presented here where Colombia qualifies and Paraguay is eliminated.

3. Who will be the 5 qualified teams?

Mathematics indicates that Argentina, Chile and Peru are the candidates to fight for the remaining slots. Who will take their songs to the World Cup? Will Chile sing, “prepárate Rusia que aquí vamos por más”? Or Peru will shout, “porque yo creo en ti, ¡vamos vamos Perú!” Or it will be Argentina who will say, “esta barra quilombera no te deja de alentar”. The total qualifying probability for each team, before the last two dates, is:

Table V: Probability to qualify in the top 5.

With the best goal difference	With the worst goal difference
P() = 190/243 = 78.2% P() = 184/243 = 75.7% P() = 144/243 = 59.3% P() = 29/243 = 11.9%	P() = 153/243 = 62.9% P() = 151/243 = 62.1% P() = 100/243 = 41.2% P() = 17/243 = 6.9%

The results given in Table V were obtained through an analysis similar to the previously developed tree diagram, for this we used the data from Tables A.2, B.2, C.2, D.2, E.2, F. 2, G.2, H.2, I.2 in the supplementary information. The interesting thing is that despite the fact that Argentina and Peru have the same number of points before playing their games, once they are finished, Peru's probability of qualifying is higher. This is explained because Argentina plays with direct rivals on both dates, while Peru on the last date does so with an “already qualified” team. Another interesting detail is to see how it affects having the best goal difference. Chile turns out to be the most benefited with it, increasing its qualifying probability by 18.1 percentage points just by scoring more goals. In contrast, Peru and Argentina increase their qualifying probability by 15.3 and 13.6 percentage points, respectively. Therefore, to make use of this advantage, the Chileans should focus on beating Ecuador at home and avoiding a massacre against Brazil. In any case, Chile has the lowest qualifying probability (excluding Ecuador), even when considering the case in which it can beat Brazil. If we consider that these results are decisive, we can conclude that the 5 qualified teams will be: Brazil, Uruguay, Colombia, Peru and Argentina. The pending question would be, which team is meant to play the intercontinental playoffs (fifth position in the standings)? Peru or Argentina? Carrying out an analysis similar to the previous one, we have:

Table VI: Probability to qualify in the top 4.

With the best goal difference	With the worst goal difference
P() = 121/243 =  49.8% P() = 121/243 =  49.8%	P() = 83/243 = 34.2% P() = 81/243 = 33.3%

Argentina gets a slightly better chance of qualifying directly to the World Cup. The reason is the same as the previous case, i.e., Argentina faces direct opponents, which becomes relevant in sub-group F, see the supplementary information. If this slight advantage were a determining factor, Peru would end up facing New Zealand for the intercontinental playoffs. The peruvian team led by Gareca would get on the plane and make the most bizarre trip of their lives. They would cross the Pacific Ocean to reach New Zealand on a trip that the clock from Santiago would tell them that it was 12 but it would actually take them 27 hours to get there and the return from Auckland would be done with the clock moving counterclockwise, but that wouldn't matter... because Spain 82' could finally be left behind.

Afternote: Peru played the intercontinental playoffs, while Brazil, Uruguay, Argentina and Colombia, directly qualified to the World Cup.