A summary of this article with some extra info appears in the following video:

There are 6 matches left to set the 32 teams that qualify to the 2018 FIFA World Cup. 4 of them are played in Europe, the other two are matches between Confederations. What teams will win? Would mathematics give us a clue? To answer this, we are going to find a correlation between the FIFA ranking and the results of the intercontinental playoffs. The FIFA ranking is a mathematical model that is described in the following article: “Understanding the mathematical model FIFA ranking for the period 2006-2018,” and it allows us to define a ranking for the teams in competition. First, we will find the correlation in previous World Cups and then from the data obtained we will project the possible outcomes for the 2018 FIFA World Cup.

Korea-Japan 2002

Table I: Intercontinental playoffs (2001).

Winner (position)		Loser (position)
Uruguay (23)	vs	Australia (48)
Ireland (20)	vs	Iran (35)
Germany (14)	vs	Ukraine (44)
Turkey (34)	vs	Austria (53)
Slovenia (44→27)	vs	Romania (14→15)
Belgium (30→33)	vs	Czech Republic (7→10)

In Table I, the position is set based on the FIFA ranking. As we can see, Uruguay, Ireland, Germany and Turkey qualified to the World Cup, defeating teams that were below them in the ranking. Slovenia and Belgium were the surprises, defeating teams that were better positioned in the ranking. For the latter two matches, we show in parentheses the evolution of the teams in the ranking. For example, Slovenia reached its final match against Romania improving its position from 44^th to 27^th. These results allow us to conclude that in 4 out of 6 games the best positioned in the ranking won, while in 2 out of 6 there were surprises.

Germany 2006

Table II: Intercontinental playoffs (2005).

Winner (position)		Loser (position)
Spain (8)	vs	Slovakia (45)
Czech Republic (3)	vs	Norway (37)
T. and Tobago (53)	vs	Bahrein (55)
Australia (57→54)	vs	Uruguay (25→17)
Switzerland (42→38)	vs	Turkey (12→11)

In Table II, the position is set based on the FIFA ranking. As we can see, Spain, Czech Republic, and Trinidad and Tobago qualified to the World Cup, defeating teams that were below them in the ranking. Australia and Switzerland were the surprises, defeating teams that were better positioned in the ranking. For the latter two matches, we show in parentheses the evolution of the teams in the ranking. For example, Switzerland reached its final match against Turkey improving its position from 42^nd to 38^th. These results allow us to conclude that in 3 out of 5 games the best positioned in the ranking won, while in 2 out of 5 there were surprises.

South Africa 2010.

Table III: Intercontinental playoffs (2009).

Winner (position)		Loser (position)
France (9)	vs	Ireland (34)
Portugal (10)	vs	B. and Herzegovina (42)
Uruguay (25)	vs	C. Rica (43)
Greece (16)	vs	Ukraine (22)
N. Zealand (99→83)	vs	Bahrein (65→61)
Slovenia (60→49)	vs	Russia (6→12)

In Table III, the position is set based on the FIFA ranking. As we can see, France, Portugal, Uruguay and Greece qualified to the World Cup, defeating teams that were below them in the ranking. New Zealand and Slovenia were the surprises, defeating teams that were better positioned in the ranking. For the latter two matches, we show in parentheses the evolution of the teams in the ranking. For example, Slovenia reached its final match against Russia improving its position from 60^th to 49^th. These results allow us to conclude that in 4 out of 6 games the best positioned in the ranking won, while in 2 out of 6 there were surprises.

Brazil 2014

Table IV: Intercontinental playoffs (2013).

Winner (position)		Loser (position)
Portugal (14)	vs	Sweden (25)
Croatia (18)	vs	Iceland (46)
Greece (15)	vs	Romania (29)
Uruguay (6)	vs	Jordan (70)
Mexico (24)	vs	N. Zealand (79)
France (21)	vs	Ukraine (20)

In Table IV, the position is set based on the FIFA ranking. As we can see, Portugal, Croatia, Greece, Uruguay, and Mexico qualified to the World Cup, defeating teams that were below them in the ranking. France was the surprise, defeating Ukraine, which was better positioned in the ranking. These results allow us to conclude that in 5 out of 6 games the best positioned in the ranking won, while in 1 out of 6 there were surprises.

The results given in the upper tables allow us to conclude that the surprises are given by teams that improved in the FIFA ranking. Therefore, for the 2018 FIFA World Cup, surprises should meet the same condition. Moreover, in each World Cup there is a tendency to have at most 2 surprises in the intercontinental playoffs. With this information we proceed to analyze the results of the intercontinental playoffs for the 2018 FIFA World Cup. To determine the teams that improved in the ranking, we write down the ranking evolution in the interval January-October 2017. The teams to consider are Sweden, Denmark, Croatia, Switzerland, Australia, Peru, Italy, Ireland, Greece, Northern Ireland, Honduras and New Zealand:

Table V: FIFA ranking (2017).

Month
January	41	47	14	11	44	19	16	23	42	32	75	109
February	44	49	16	11	54	18	15	25	43	35	65	111
March	45	48	16	11	55	18	15	24	46	35	65	112
April	34	51	18	9	50	17	12	26	39	26	68	112
May	34	51	18	9	50	17	12	26	39	26	68	112
June	34	51	18	9	48	15	12	26	40	28	69	95
July	18	47	15	5	45	14	12	29	38	22	72	122
August	19	46	16	4	45	15	12	29	38	23	75	123
September	23	26	18	17	50	12	17	34	47	20	74	113
October	25	19	18	11	43	10	15	26	47	23	69	122

We then plot the data given in Table V. We fit these data to a line using the least squares method. A more advanced study of this method is given in the tutorial: "The least squares method with Despacito." This linear fit is what we call a linear trend because we are approximating a given data to a line in order to easily deduce if over time a team improved in the FIFA ranking or not. The lines with a negative slope indicate an improvement in the ranking. These calculations were developed in Python, you can download the code from my repository @Github.

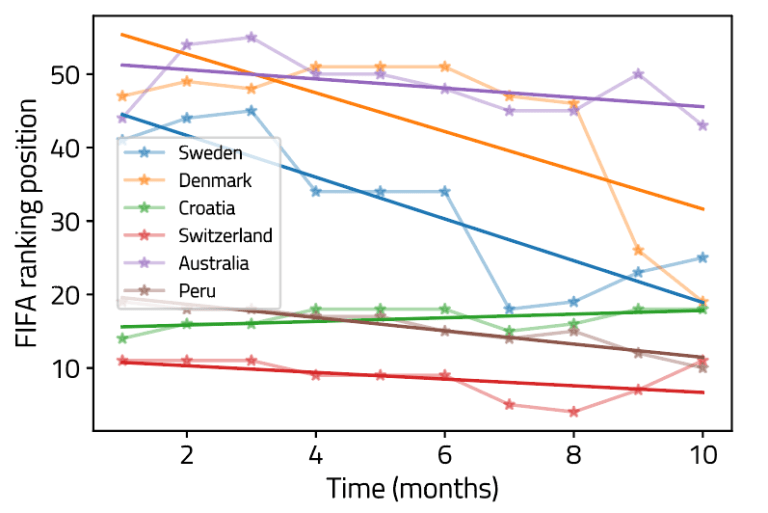
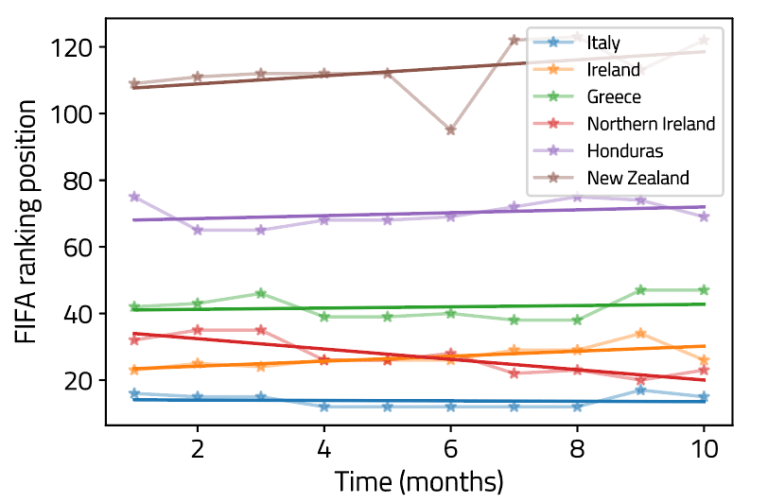
In Figs. 1 and 2 we can see that the teams that have had a good evolution in the ranking throughout 2017 are Sweden, Denmark, Switzerland, Australia, Peru and Northern Ireland; therefore, it is plausible to think that they have a better chance to qualify to the World Cup. Let’s now see the matches to be played:

Tabla VI: Intercontinental playoffs (2017).

Winner (position)		Loser (position)
Peru (10)	vs	N. Zealand (122)
Australia (43)	vs	Honduras (69)
Denmark (19)	vs	Ireland (26)
Croatia (18)	vs	Greece (47)
Switzerland (11)	vs	Northern Ireland (23)
Sweden (25)	vs	Italy (15)

Peru ranked 10^th faces New Zealand ranked 122^th. Considering that Peru reaches its final game with a good evolution in the FIFA ranking, then we can conclude that Peru will win its match. The same conclusion can be obtained with Australia and Denmark; therefore, these three teams will undoubtedly emerge victorious.

Three encounters remain to be analyzed. Remember that at most there must be 2 surprises, that is, in the worst case, out of the three remaining matches, only in one will the one who is best positioned in the FIFA ranking win. Although Croatia has not evolved positively in the ranking, its chances of qualifying to the World Cup are high because it finished in position 18^th while its rival Greece, who also did not evolve positively, finished in position 47^th. Therefore, we are going to conclude that Croatia qualifies. Therefore, surprises may only come from the matches Switzerland vs Northern Ireland and Sweden vs Italy. While Northern Ireland has improved over time, unluckily for them, Switzerland has also improved, and therefore it is more feasible to think that Switzerland will qualify. Therefore, the only surprise will be given in the Sweden vs Italy match, where Sweden has improved greatly throughout 2017, while Italy has stalled in the 15^th position. Consequently, our analysis suggests that those who qualify to the World Cup will be: Peru, Australia, Switzerland, Croatia, Denmark and Sweden.

Afternote: The aforementioned teams qualified to 2018 FIFA World Cup.